Definition/Introduction
Throughout life, the production and loss of heat are constantly balanced. After death, the body cools down because its metabolism and heat production stop. The deceased body loses heat through conduction, convection, radiation, and evaporation.
Definition
Algor mortis (translated from Latin: Algor = "coldness" and Mortis = "of death") is defined as the internal cooling of the body after death to ambient temperature due to the cessation of thermoregulation. This process is the propensity of a body to reach equilibrium with ambient temperature after death.
The term algor mortis was coined by Bennet Dowler in 1849.[1] The first recorded measurements of the postmortem temperature intervals were made by John Davy in 1839.
After death, individuals no longer produce body heat or cooling mechanisms, and the decedent's temperature slowly approaches ambient temperature. This variable is based on the assumption that body temperature was normal at the time of death and includes both temperatures above and below normal living body temperature, 98.7 °F. Rectal temperatures are commonly used as the standard to determine the decedent temperature and algor mortis.[2]
Determining the time of death is crucial in forensic investigations. Algor mortis is one of the earliest and most widely used phenomena to estimate the time since death or postmortem interval (PMI). This phenomenon is one of the three cardinal signs of death, the other ones being livor mortis and rigor mortis. Algor mortis is frequently mentioned in forensic literature and portrayed in the media; however, its accuracy as a PMI indicator varies greatly. The physiological underpinnings of algor mortis, variables affecting body cooling, and the constraints of its forensic uses—particularly in refrigerated and cold environments—are examined in this activity. Furthermore, novel research approaches and technological developments are required to enhance the precision of PMI estimation.
Mechanisms
Estimating the PMI is challenging due to many intrinsic and extrinsic factors that affect cooling rates, even if the theory is straightforward. Forensic pathologists must comprehend these factors to produce a more precise time of death diagnosis.
Throughout life, the hypothalamus regulates body temperature to maintain an internal temperature of approximately 36.9 °C (98.6 °F). Heat dissipation results from the body's inability to maintain this equilibrium after death. Due to residual metabolic activity, the cooling rate initially slows before gradually following a sigmoid curve. The core temperature stays constant for several hours before dropping steadily over the next 12 hours, often at a pace of 1 to 1.5 °C/h. However, environmental factors have a significant impact on this rate. Notably, ambient conditions such as humidity, air circulation, and closeness to heat sources can significantly impact the cooling rate.
Factors Affecting the Rate of Cooling
- Environmental temperature: Cooling occurs more quickly when a larger temperature differential exists between the body and its environment. Although severely cold conditions can produce freezing and change the pace of temperature loss, high heat may delay cooling.
- Body composition: Subcutaneous fat and a higher body mass index (BMI) slow down cooling rates by insulating heat. On the other hand, leaner bodies with less body fat shed heat more quickly. Studies have shown that the BMI influences cooling rates, but only 36% of cases were in a linear progression over time, even in a controlled ambient temperature.[3]
- Clothes and coverings: Although wet clothing speeds up cooling, clothing traps heat, minimizing heat loss. Another critical factor in determining the pace of heat dissipation is the type and thickness of garment materials.
- Body position and exposure: Heat loss is enhanced by exposure to flowing air or water; a stretched-out body cools more quickly than a curled one. Because water has a profound thermal conductivity, submersion in it can result in a significantly altered cooling pattern.
- Surface contact: When the body comes into contact with a cold surface, conductive heat loss rises. In contrast to wood or carpeted flooring, metal and stone surfaces tend to dissipate heat more quickly.
- Humidity: Cooling is faster in a humid atmosphere than in a dry one.
- Age: Infants and the geriatric population generally lose body temperature more rapidly than middle-aged adults.
Methods for Measuring Postmortem Cooling
Formulas are being developed to determine the PMI more accurately for medicolegal investigations [New Jersey Institute of Technology. Improving methods to estimate time of death from body temperature ]. There are complex algorithms that take many variables to determine the PMI. The development of faster formulas based on the level of decomposition, humidity, and temperature (algor mortis) significantly impacts investigations. Some of these formulas become less accurate at higher temperatures and significantly overestimate, and cold temperatures significantly underestimate the PMI.[4] Climate variables besides ambient temperature significantly influence decedent algor mortis and decomposition rate. In some studies, developed equations for estimating PMI were 10% correct in indoor death scenes at one location and 60% at another.[5]
The most common methods for taking a core body temperature are rectally or by passing a thermometer through the upper right abdominal quadrant into the liver. The brain, tympanic membrane, and abdomen skin are other possible locations. The postmortem temperature changes have been extensively studied using nomograms, which are diagrams representing the relationship between multiple variables that can be mathematically quantified. The Henssge nomogram, which accounts for body mass, clothing, and ambient temperature, is still the most extensively used mathematical model for PMI estimation. Infrared thermography and thermal photography are also becoming popular noninvasive techniques for determining postmortem body temperature loss.
The corpse's huge thermal mass causes the rectal temperature to decline more slowly in the initial hours following death, resulting in a sigmoidal temperature distribution (double exponential curve or flipped S) and the so-called postmortem plateau.
Common Formulas for PMI Estimation
The Glaister equation:
The Glaister equation estimates the PMI based solely on algor mortis. The equation is expressed as:
PMI = (98.7 °F − rectal temperature of the decedent (°F)) / (T)
where:
- T = 1.5 if ambient temperature < 32 °F.
- T = 0.75 if ambient temperature ≥32 °F.
This formula assumes an approximate cooling rate of 1.5 °F/h under typical conditions.
Henssge Nomogram:
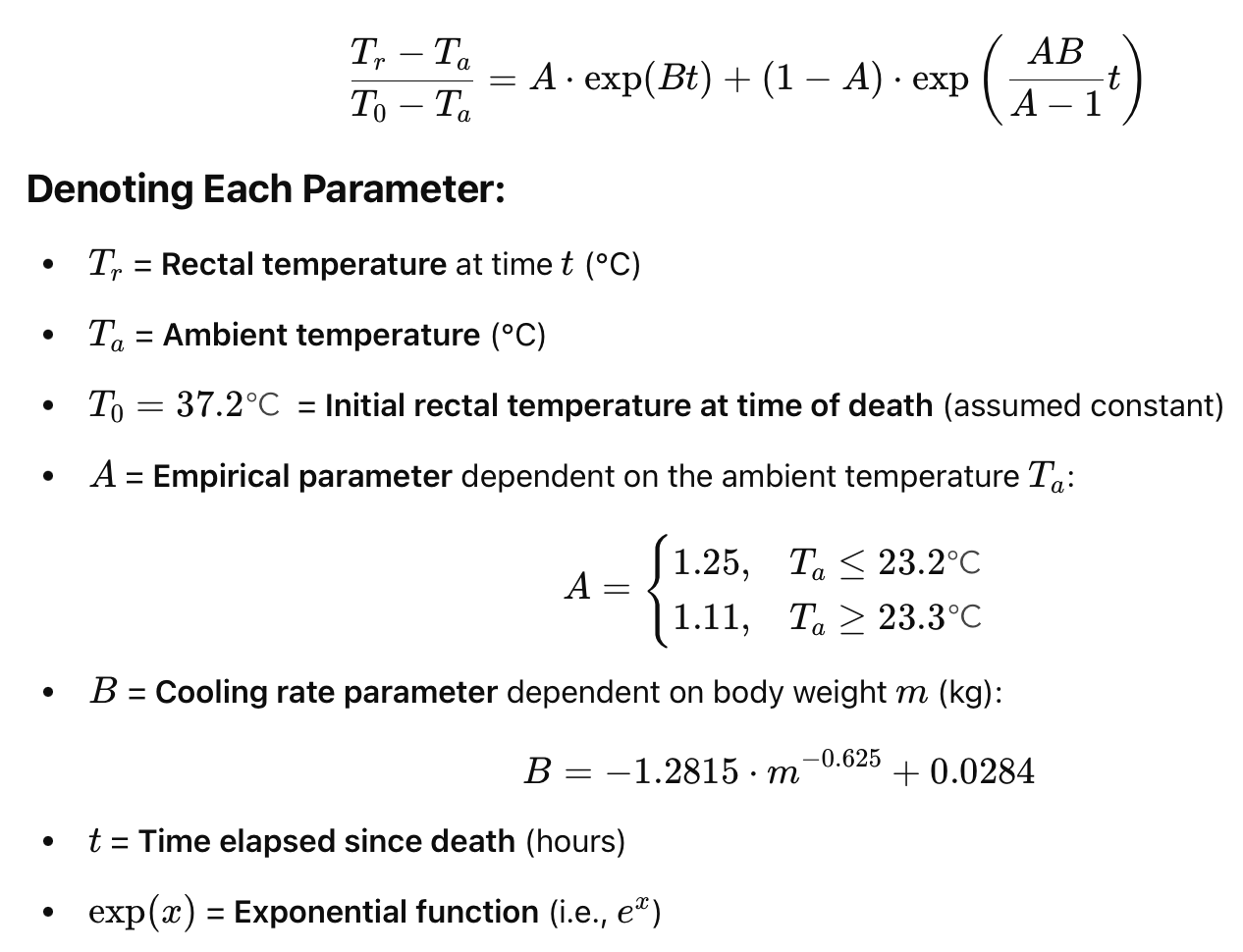
Henssge nomogram is the most precise method for estimating the PMI by measuring temperature [ScienceDirect. Human Body Decomposition]. This method is most accurate within the first 10 hours post-mortem.[6] The usual nomogram does not account for sudden changes in ambient temperature, such as when bringing the cadaver into a cooling chamber. In contrast, the Henssge nomogram relies on repetitive examinations of body cooling behavior under different conditions.[7] In addition to temperature, the method considers variables such as body weight, clothing, and whether the body is in air or water. The Henssge formula is a mathematical formula used to describe the trends observed in the nomogram (see Image. The Henssge Formula).
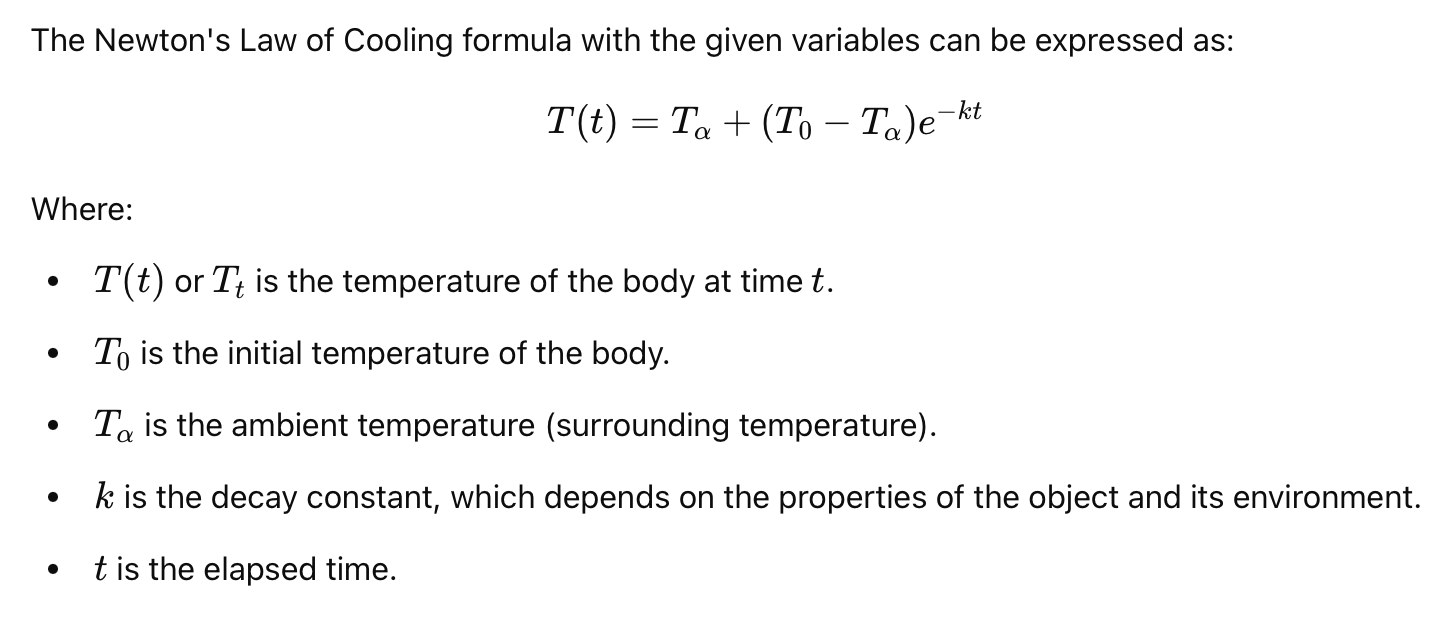
Newton's law of cooling:
Newton's law of cooling states that the rate at which the temperature of an object cools is proportional to the difference in temperature between the object and its surroundings (see Image. Newton's Law of Cooling). If someone leaves coffee at 70 °C at a room temperature of 20 °C, the temperature of the coffee decreases to 60 °C and then 50 °C over time. Because of metabolic and environmental variables, it may not always apply completely to human cadavers, although it offers a theoretical model for heat dissipation.
Issues of Concern
Register For Free And Read The Full Article
Search engine and full access to all medical articles
10 free questions in your specialty
Free CME/CE Activities
Free daily question in your email
Save favorite articles to your dashboard
Emails offering discounts
Learn more about a Subscription to StatPearls Point-of-Care
Issues of Concern
Limitations of Algor Mortis in Forensic Investigations
Although algor mortis offers a general framework for estimating PMI, several inherent limitations affect its accuracy:
- Differences in initial body temperature: The assumption that all individuals die with a body temperature of 98.6 °F is flawed. Factors such as fever, hypothermia, or drug use can significantly alter the starting temperature.
- Influence of external factors: Variability is significantly increased by wind, submersion in water, and clothing layering.
- Effect of postmortem refrigeration: Research shows that bodies that have been refrigerated show a linear cooling pattern instead of a plateau phase, which makes standard equations less reliable.
- PMI estimation accuracy: The method is unreliable for extended PMI estimations because the margin of error increases significantly after 12 hours post-mortem.
- Decomposition: As decomposition proceeds, heat may be produced by gas formation and bacterial activity, which can interfere with regular cooling cycles.
Postmortem Caloricity
Postmortem caloricity is a condition in which the body's temperature rises instead of decreasing after death.
The causes of postmortem caloricity include:
- A corpse left out in the open during warm summers
- Infections—such as cholera, malaria, septicemia, tetanus, and typhoid—may have increased temperature at the time of death, and the metabolism of bacteria and other microorganisms continues after death.
Clinical Significance
The assessment of algor mortis changes alone is insufficient in determining the PMI. However, it remains an important variable in defining equations and formulas to estimate the time of death in medicolegal death investigations.
In cases where sexual assault is suspected, caution should be exercised to avoid inserting a thermometer into the rectum before collecting trace evidence.
Nursing, Allied Health, and Interprofessional Team Interventions
Recent Research
Several studies have examined the relationship between BMI and cooling rates, showing that obese individuals cool more slowly than individuals of average weight. Although other variables, such as attire and environmental exposure, are essential, regression analysis indicates a moderate relationship between BMI and cooling rate. In forensic pathology, applying machine learning models and predictive algorithms to improve PMI estimates based on various criteria is becoming more popular.[8]
Furthermore, refrigerated bodies challenge conventional PMI estimate techniques by deviating from typical cooling curves. The results from different studies highlight that although algor mortis might offer broad temporal periods, it should not be used as the only indicator of PMI. Future research should look into combining entomological data with biochemical markers to improve PMI's dependability.
Conclusion
Although algor mortis is still a fundamental idea in forensic science, it is difficult to put it into practice when estimating PMI. Because of its intrinsic unpredictability, it may provide initial assistance in forensic casework, but for a more exact calculation of time since death, additional approaches such as livor mortis, rigor mortis, and forensic entomology are required. PMI calculations could be improved with the ongoing development of forensic technology, such as artificial intelligence–based predictive modeling and thermal imaging. To improve the precision of PMI calculations, future studies should concentrate on enhancing predictive models by integrating machine learning methods and larger datasets.
Media
(Click Image to Enlarge)
References
RIESS K. The rebel physiologist, Bennet DOWLER. Journal of the history of medicine and allied sciences. 1961 Jan:16():39-48 [PubMed PMID: 13741567]
Igari Y, Hosokai Y, Funayama M. Rectal temperature-based death time estimation in infants. Legal medicine (Tokyo, Japan). 2016 Mar:19():35-42. doi: 10.1016/j.legalmed.2016.02.002. Epub 2016 Feb 1 [PubMed PMID: 26980252]
Wardak KS, Cina SJ. Algor mortis: an erroneous measurement following postmortem refrigeration. Journal of forensic sciences. 2011 Sep:56(5):1219-21. doi: 10.1111/j.1556-4029.2011.01811.x. Epub 2011 Jun 3 [PubMed PMID: 21644987]
Cockle DL, Bell LS. Human decomposition and the reliability of a 'Universal' model for post mortem interval estimations. Forensic science international. 2015 Aug:253():136.e1-9. doi: 10.1016/j.forsciint.2015.05.018. Epub 2015 May 27 [PubMed PMID: 26092190]
Maile AE, Inoue CG, Barksdale LE, Carter DO. Toward a universal equation to estimate postmortem interval. Forensic science international. 2017 Mar:272():150-153. doi: 10.1016/j.forsciint.2017.01.013. Epub 2017 Jan 18 [PubMed PMID: 28183035]
Henssge C. Death time estimation in case work. I. The rectal temperature time of death nomogram. Forensic science international. 1988 Sep:38(3-4):209-36 [PubMed PMID: 3192144]
Level 3 (low-level) evidenceBovenschen M, Schwender H, Ritz-Timme S, Beseoglu K, Hartung B. Estimation of time since death after a post-mortem change in ambient temperature: Evaluation of a back-calculation approach. Forensic science international. 2021 Feb:319():110656. doi: 10.1016/j.forsciint.2020.110656. Epub 2020 Dec 15 [PubMed PMID: 33373761]
Dani LM, Tóth D, Frigyik AB, Kozma Z. Beyond Henssge's Formula: Using Regression Trees and a Support Vector Machine for Time of Death Estimation in Forensic Medicine. Diagnostics (Basel, Switzerland). 2023 Mar 27:13(7):. doi: 10.3390/diagnostics13071260. Epub 2023 Mar 27 [PubMed PMID: 37046478]